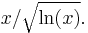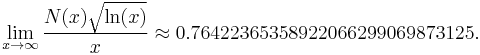Landau–Ramanujan constant

In mathematics, the Landau–Ramanujan constant occurs in a number theory result stating that the number of positive integers less than x that are the sum of two square numbers, for large x, varies as

$x/{\sqrt{\ln(x)}}.$

The constant of proportionality is the Landau–Ramanujan constant, which was discovered independently by Edmund Landau and Srinivasa Ramanujan.

More formally, if N(x) is the number of positive integers less than x which are the sum of two squares, then

$\lim_{x\rightarrow\infty} \frac{N(x)\sqrt{\ln(x)}}{x}\approx 0.76422365358922066299069873125.$

External links